Lesson Progress
0% Complete
Topic Content:
- Addition & Subtraction in Other Bases
Worked Example 2.3.1:
Solve the following:
a. 3042five + 2103five
b. 2001three + 1022three
c. 151.24six + 12.34six + 20.11six
d. 53468 – 6378
e. 3002four – 2213four
Solution
a. 3042five + 2103five
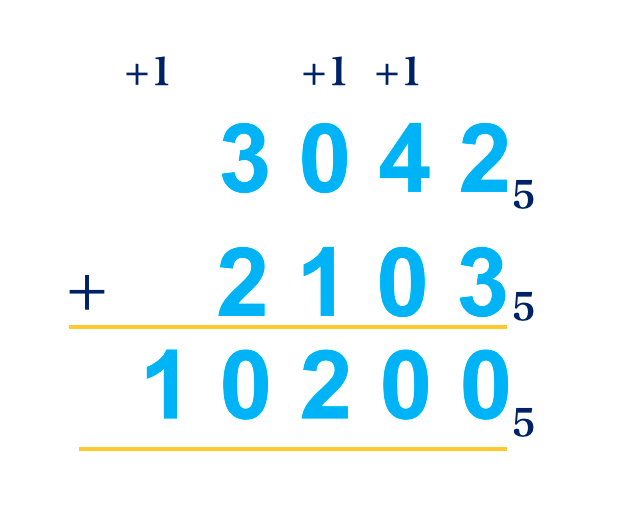
Explanation:
1st Column (from right to left): 2 + 3 = 5. How many fives are there in 5? \( \frac{5}{5} \)= 1 remainder 0. Write 0 and carry 1 to column 2.
You are viewing an excerpt of this Topic. Subscribe Now to get Full Access to ALL this Subject's Topics and Quizzes for this Term!
Click on the button "Subscribe Now" below for Full Access!
Subscribe Now
Note: If you have Already Subscribed and you are seeing this message, it means you are logged out. Please Log In using the Login Button Below to Carry on Studying!
🤓🤓🤓🤓🤓🤓🤓🤓🤓🤓🤓🤓BRAINSTORMING!!!