Equations Involving Number Bases

In this topic we will be guiding you through the necessary steps required to solve problems related to Number Bases.

Worked Example 2.6.1:

If 132_four = X_eight  find X

Solution

First convert the left hand side (132_four ) to base 10

132_four  = 1 × 4² + 3 × 4¹ + 2 × 4⁰

16 + 12 + 2 = 30_ten

Since 30_ten = X _{eight ,}  Find the value of X.

convert 30_ten to base eight

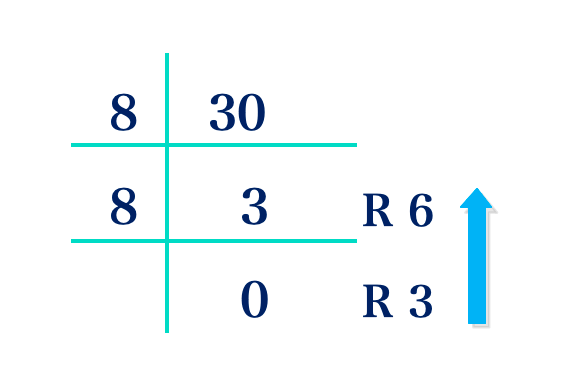
∴ 30_ten = 36_eight

30_ten = X_eight

∴ 36_eight = X_eight

Answer: X = 36

Worked Example 2.6.2:

43_x =  11111_two , Find x

Solution

convert both sides to base 10

(4 × x¹ + 3 × x⁰)_ten   = 1 × 2⁴ + 1 × 2³+ 1 × 2² + 1 × 2¹ + 1 × 2⁰