Lesson Progress
0% Complete
Topic Content:
- Application of Cosine Rule
Example 6.4.1:
Solve the triangle in which a = 4.5 m, b = 5.3 m, and C = 112°
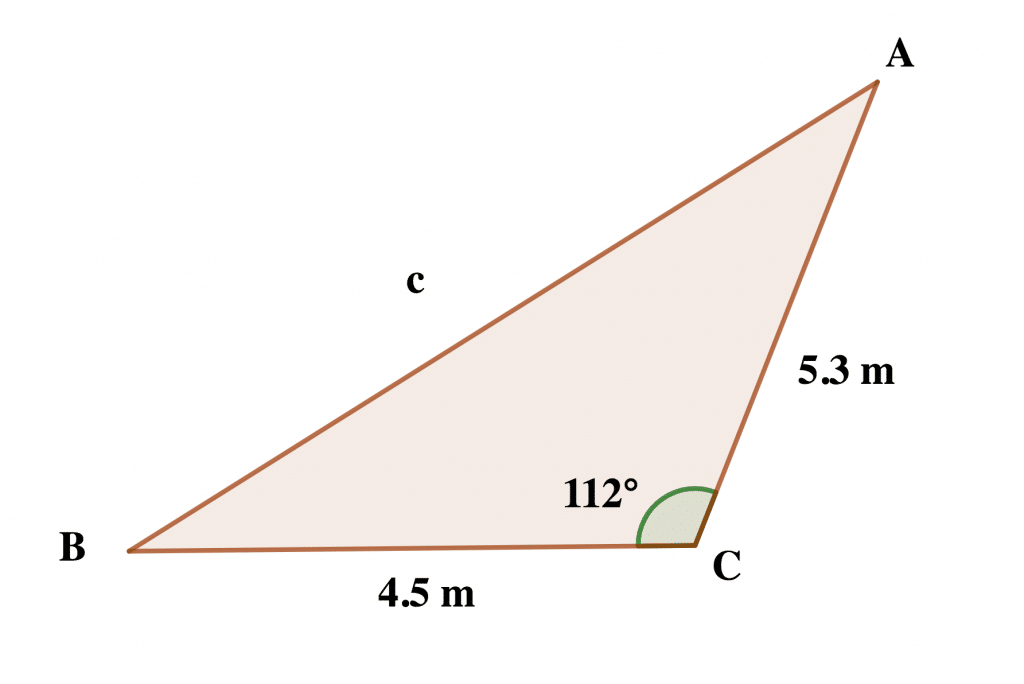
Solution:
To find length c we will use the cosine rule formula.
You are viewing an excerpt of this Topic. Subscribe Now to get Full Access to ALL this Subject's Topics and Quizzes for this Term!
Click on the button "Subscribe Now" below for Full Access!
Subscribe Now
Note: If you have Already Subscribed and you are seeing this message, it means you are logged out. Please Log In using the Login Button Below to Carry on Studying!
Responses